


Have you ever wondered how trigonometry, the branch of mathematics dealing with triangles, fits into your daily life beyond textbook problems and exams? At first glance, it may seem like just another academic subject filled with angles, formulas, and functions. But in reality, trigonometry is a powerful tool with countless applications in the real world.
From designing tall buildings to crafting your favorite video games, trigonometry quietly supports our modern world. It helps pilots navigate, engineers build bridges, and even artists create realistic perspectives. Understanding how trigonometry works in practical settings can give you a whole new appreciation for the subject—and it might even inspire you to enjoy math more!
This blog will break down the core concepts of trigonometry, showcase real-life applications across various fields, provide relatable examples, and share helpful tips for mastering the subject. By the end, you’ll see why trigonometry is not just about triangles—it’s about solving real-world problems with precision and creativity.
Before we dive into how trigonometry is used in the real world, let's take a moment to understand its core principles. Once you understand the basics, the more advanced applications will make a lot more sense.
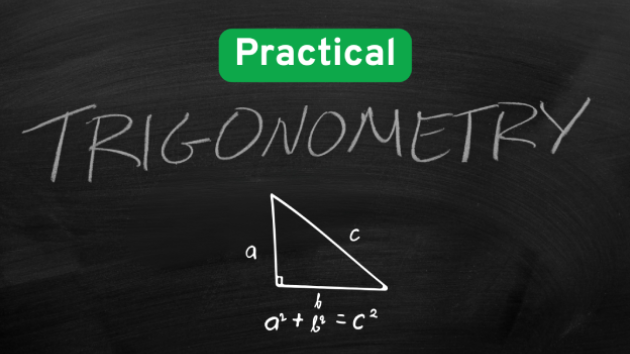
Trigonometry is the branch of mathematics that studies the relationships between the sides and angles of triangles—particularly right-angled triangles. At its core, it’s all about understanding how these parts relate to one another.
The heart of trigonometry lies in three essential functions: sine (sin), cosine (cos), and tangent (tan). These functions compare different sides of a right triangle.
For example, if a triangle has:
Then:
These ratios help solve for unknown angles or side lengths in problems involving right triangles.
The unit circle is a critical concept in trigonometry. It’s a circle with a radius of one unit, centered at the origin (0,0) on a graph. The unit circle helps you understand how sine, cosine, and tangent behave as angles increase or decrease.
For example, at 0° (or 0 radians), the cosine value is 1, and the sine is 0. These patterns repeat every 360° or 2π radians, which is why trigonometry is so helpful in modeling cycles and waves.
Trigonometry also includes three reciprocal functions:
These are useful in more advanced applications such as physics, engineering, and calculus.
Now that we understand the basics, let’s explore where trigonometry makes an impact in the real world. You might be surprised by how often it's used!
Trigonometry plays a crucial role in navigation. Pilots, ship captains, and even astronauts use trigonometric functions to calculate distances, angles, and directions. GPS systems rely on satellite signals and trigonometric calculations to determine your exact location on Earth.
When navigating from one location to another, trigonometry helps calculate the shortest path or the optimal direction. That’s why your phone's map app can tell you exactly how far and how long your trip will be.
In construction and design, trigonometry is a must-have tool. Engineers use it to calculate the forces acting on structures, while architects use it to design buildings with specific shapes and angles.
For example:
Even the strength and durability of a building depend on accurate trigonometric calculations.
Astronomers use trigonometry to measure distances between stars and planets. By applying parallax (the apparent shift in position of a star due to Earth’s movement), scientists can use triangles to estimate how far away celestial objects are.
NASA and other space agencies also use trigonometry to calculate rocket trajectories and satellite orbits.
Physics is filled with trigonometric concepts. Sound waves, light waves, and electromagnetic waves are often represented as sine or cosine functions.
For example:
Understanding these principles helps us build better audio devices, power grids, and communication systems.
Sports analysts use trigonometry to improve performance and strategies. For example:
The Statcast system in Major League Baseball tracks player movements and ball trajectories using trigonometry and physics.
Game developers use trigonometry to design realistic movements and graphics. Whether it’s a car turning a corner, a character jumping, or a camera panning across the scene, trigonometric functions ensure the motion looks smooth and accurate.
Without trigonometry, 3D game environments would look flat and unnatural.
Construction professionals use trigonometry every day to:
For example, when building a wheelchair-accessible ramp, the angle must meet legal standards. Trigonometry helps calculate the appropriate incline to ensure safety and functionality.
Even in situations that seem unrelated to math, trigonometry can still be found.
Sound engineers use sine and cosine waves to manipulate audio frequencies. Equalizers, synthesizers, and digital sound effects rely on trigonometric models to fine-tune the audio experience.
When you listen to music on your headphones, trigonometry helps deliver clear, balanced sound.
Trigonometry is essential in understanding perspective in art. When drawing a scene with depth—like a road disappearing into the horizon—artists use angles to represent space realistically.
Photographers use trigonometry to calculate depth of field, focal length, and lighting angles to capture stunning images.
Trigonometry is also used in crime scene investigations. For example, if a bullet hole is found in a wall, forensic experts can calculate the angle of entry to determine the shooter's location. Blood spatter analysis also relies on trigonometry to estimate points of origin.
Trigonometry can feel intimidating, but with the right approach, anyone can master it. Here are some tips and resources to make your journey easier:
Use diagrams and visuals to understand how angles and sides relate. Drawing triangles and labeling them helps you grasp concepts more clearly.
Spend time memorizing the unit circle values (like sin(30°) = 0.5). This will make solving problems quicker and easier.
Trig identities like:
…are essential tools for solving complex equations. Practice applying them in different problems.
There are many helpful tools out there:
Real-world problems strengthen your problem-solving skills. Try to solve questions that ask for heights, distances, or angles in real-life scenarios.
Strong algebra skills help in understanding trigonometry. Make sure you're comfortable with fractions, square roots, and basic equations.
As with any skill, practice is the key to mastering trigonometry. Solve a variety of problems, review your mistakes, and keep challenging yourself with harder problems.
Trigonometry isn’t just for students or mathematicians—it’s a real-world skill that impacts everything from building design to digital entertainment. Once you begin to recognize its role in everyday life, you’ll find it’s not only useful but also fascinating.
Understanding trigonometry opens up opportunities in science, technology, engineering, art, and beyond. Whether you dream of becoming an architect, a game developer, a pilot, or a musician, a solid foundation in trigonometry can help you get there.
So the next time someone says, “When will I ever use this in real life?”—you’ll have plenty of answers.
At PALS Learning Center, we believe that every student has the potential to succeed in math—with the right support. Whether your child is just being introduced to trigonometry or is preparing for advanced applications in physics, engineering, or standardized tests, our experienced tutors provide personalized instruction that breaks down complex topics into understandable steps. We make math engaging by connecting it to real-world scenarios—just like the examples in this blog—so students not only learn but also appreciate the power of what they're studying.
If your child is struggling with trigonometric concepts or wants to gain a competitive edge, we’re here to help!
👉 Contact us today to schedule a free consultation: https://palsnorthedison.com/contact-us
📚 Explore more educational blogs and resources here: https://palsnorthedison.com/blog
Let’s unlock the beauty of math—together.